EN280/13001

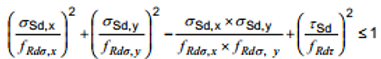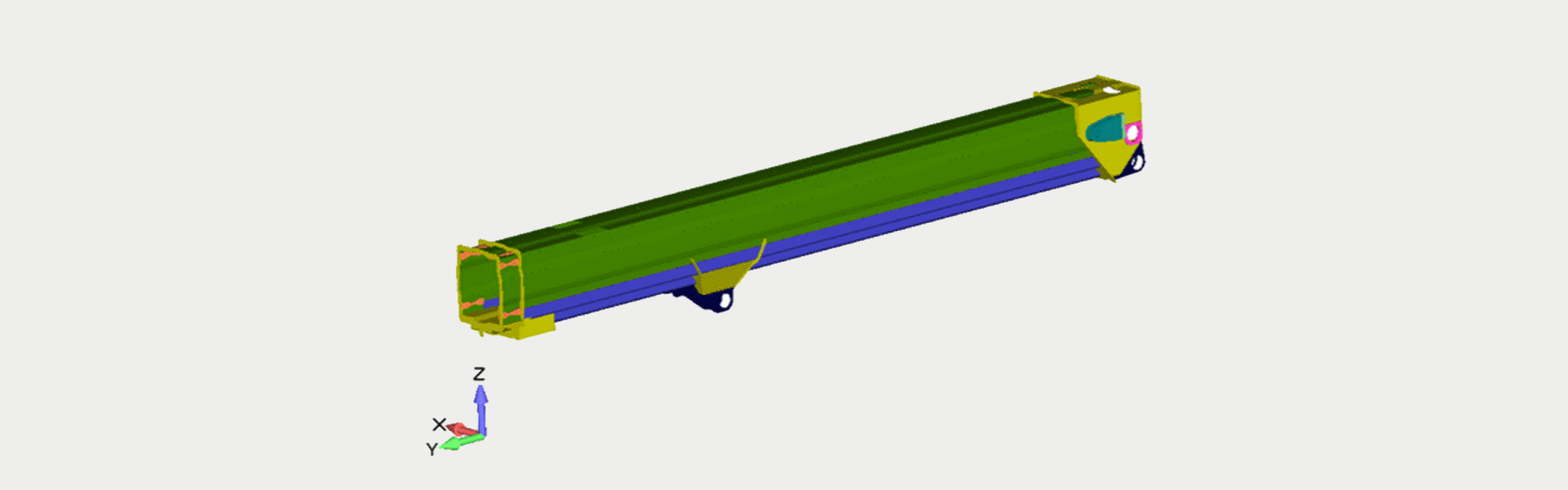
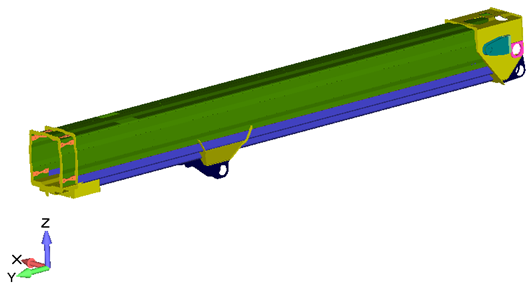
Four checks according to EN280/13001 were performed for Crane: Static strength, Static strength for welds, Fatigue check and Fatigue check for welds:

Proof of static strength

The limit design stress ƒ_Rd, used for the design of structural members, shall be calculated from:

ƒRdσ=	ƒy	for normal stress
	ϒRm
ƒRdτ=	ƒy	for shear stress
	ϒRm√3

with ϒ_Rm=ϒ_m×ϒ_sm

where

ƒy	is the minimum value of the yield stress of the material
ϒsm	is the specific resistance factor for material as follows

For non-rolled material:

ϒ_sm=0.95

For rolled materials (e.g. plates and profiles):

ϒsm=0.95	for stresses in the plane of rolling
ϒsm=0.95	for compressive and shear stresses

For tensile stresses perpendicular to the plane of rolling:

Material shall be suitable for carrying perpendicular loads and be free of lamellar defects

ϒ_sm=1.0 for plate thicknesses less than 15 mm or material in quality classes Z25 or Z35 in accordance with EN 10164:2004.

ϒ_sm=1.16 for material in quality classes Z15 in accordance with EN 10164:2004.

ϒ_sm=1.34 for materials without quality classification in accordance with EN 10164 but, conforming to classes S2 and E3 10160:1999.

ϒ_sm=1.50 without quality classification of through-thickness property.

Proof for structural members

For the structural members to be designed it shall be proven that:

σ_Sd ≤ ƒ_Rdσ and τ_Sd ≤ ƒ_Rdτ

where

σSd, τSd	are the design stresses. The von Mises equivalent stress may be used as the design stress instead.
ƒRdσ, ƒRdτ	are the corresponding limit design stresses in accordance with clause 1. I case von Misses is used, ƒRdσ is the limit design stress.

In a case of plane states of stresses when von Mises stresses are not used it shall additionally be proven that:

where

x,y	indicate the orthogonal directions of stress components.

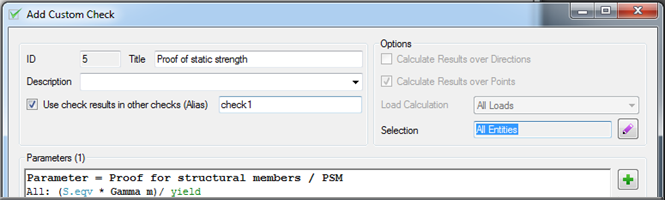
Implementation

S.eqv takes into account all stress direction.

Gamma_m – specific resistance factor for materials, which described above.

yield – yield stress of the materials.

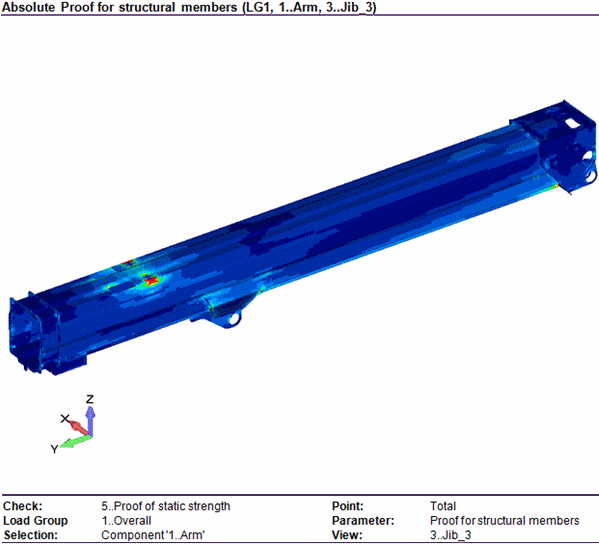
Results

On pictures below shown results of the Static check for all model. All results above 1 are unacceptable.

Proof of static strength (welds)

Depending on the equation number given in table 1, the limit design weld stress ƒ_w,Rd shall be calculated entirely by:

ƒw,Rd=	αw׃y
	ϒm

of by

ƒw,Rd=	αw׃uw
	ϒm

where

αw	is a factor given in table 1 in dependence on the type of weld, the type of stress and the material.
ƒy	is the minimum value of the yield strength of the connected members under consideration.
ƒuw	is the ultimate tensile strength of the weld material (all weld metal).

Table 1 – Factor of limit weld stress

Type of weld material	Direction of stress	Type of weld	Type of stress	equation number	ƒy≤ N/mm2	αw ƒy>420 ƒy<930 N/mm2	ƒy≥930 N/mm2
Matching (ƒy refers to the welded members)	Stress normal to the weld direction	Full penetration weld	Tension or compression	21	1.0		0.93
		Partial penetration welda	Tension or compression	21	0.90		0.85
	Stress parallel to the weld direction	all welds	Shear	21	0.60		0.55
Under-matching (ƒy refers to the weld material)	Stress normal to the weld direction	Full penetration weld	Tension or compression	22	0.80	0.85	0.90
		Partial penetration welda	Tension or compression	22	0.70	0.75	0.80
	Stress parallel to the weld direction	all welds	Shear	22	0.45	0.50	0.50

The values of α_w are valid for welds in quality C of EN ISO 5817:2007 of better.

The proof of the connected members, in accordance with 3.5.1 is always required in addition to the proof of the weld, in accordance with 3.5.4. In a case of connected members from different materials, the proof shall be made for each member separately.

For the definition of full penetration and partial penetration, weld see EN ISO 17659.

Matching welds material: weld material with ultimate strength equal or better than those of connected members.
Under matching welds material: weld material with ultimate strength less than those of connected members.

The welds joining parts of built-up members, e.g. flange-to-web connections, may be designed without regard to normal stress parallel to the axis of the weld, provided the welds are proportioned to accommodate the shear forces developed between those parts.

An asymmetric weld is not recommended, However, if used connected members shall be supported so as to avoid the effect of load eccentricity on the weld.

Proof for weld connections

for the weld to be designed it shall be proven that:

σ_w,Sd and τ_Sd ≤ ƒ_Rdσ

where

τSd, σw,Sd	are the design weld stresses.
ƒw,Rd	is the corresponding limit design weld stress.

Implementation

With a weld yield stress of 735 MPa, the allowable static stress in a weld can be summarized as follows:

Stress type	Yield connected material
	Matching	Undermatching
	below 735 Mpa	up to 930 Mpa	above 930 Mpa
S_normal	yield	0.85×735	0.9×735
T_shear	0.6×yield	0.5×735	0.5×735

Checks for weld were done for normal and shear stress type. X and Y are normal stress and XY – shear stress in welds. Also was calculated equivalent stress for all direction. On results, plots show overall results.

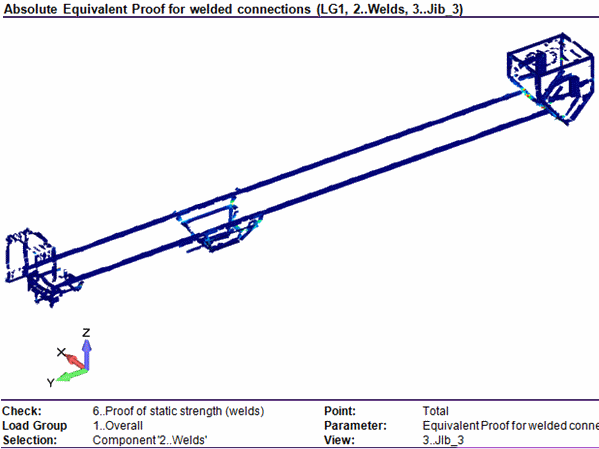
Results

On pictures below shows results of a Static check for welds. All results above 1 are unacceptable.

Fatigue check

For the detail, under consideration, it shall be proven that:

Δσ_Sd ≤ Δσ_Rd

Δσ_Sd = maxσ – minσ

where

ΔσSd	is the maximum range of design stresses.
maxσ, minσ	are the extreme values of design stresses (compression stresses with negative sign).
ΔσRd	is the limit design stress range.

The limit design stress range shall be calculated from:

ΔσRd =	Δσc
	ϒmf×3√S3

where

ΔσRd	is the limit design stress range;
Δσc	is the characteristic fatigue strength of details with m = 3;
S3	is the classified stress history parameter;
ϒmf	is the fatigue strength specific resistance factor;

For the most severe ϒ_mf = 1.25 and the values of Δσ_Rd in dependence on the class S and Δσ_c.

Implementation

Explanations for all parameters in check see above.

Results

On pictures below shown results of Fatigue check for all model. All results above 1 are unacceptable.

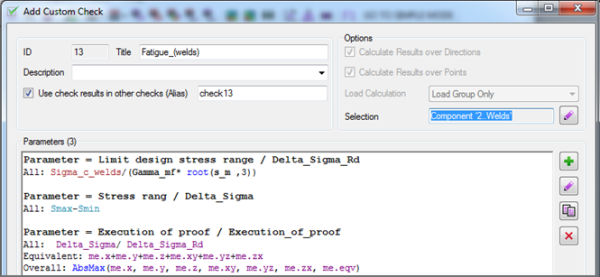
Fatigue check (welds)

Implementation

The explanation for all parameters in check see above.

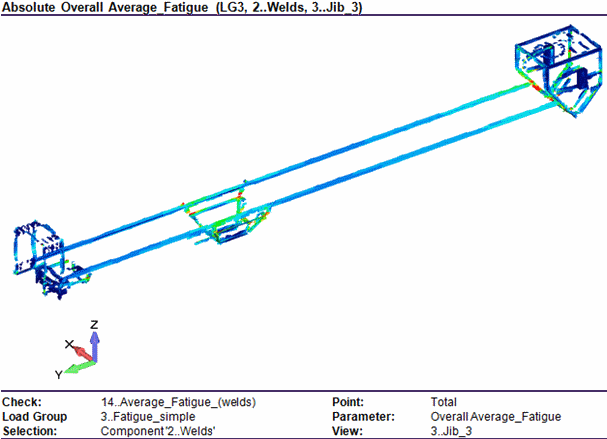
Results

On pictures below shows results of Fatigue check for welds. All results above 1 are unacceptable.